Absolute Value Inequalities

1. "Less Than" Absolute Value Inequalities

When an inequality has an absolute value we will have to remove the absolute value in order to graph the solution or give interval notation. The way we remove the absolute value depends on the direction of the inequality symbol. Recall that absolute value is defined as the distance from zero.

EXAMPLE

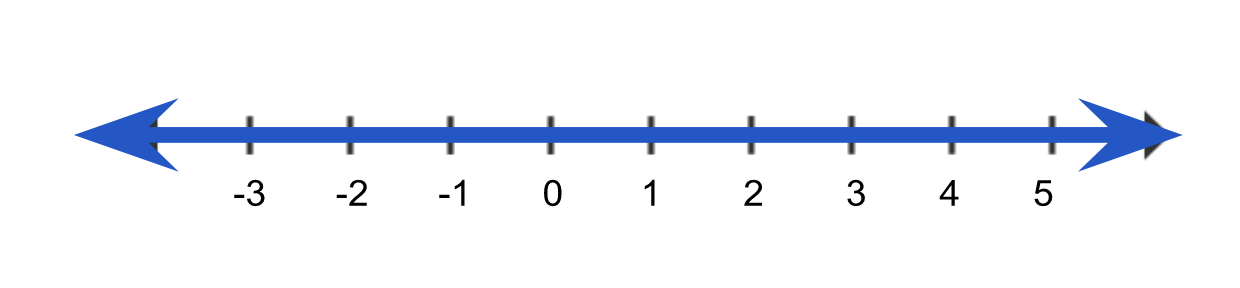
Consider .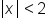

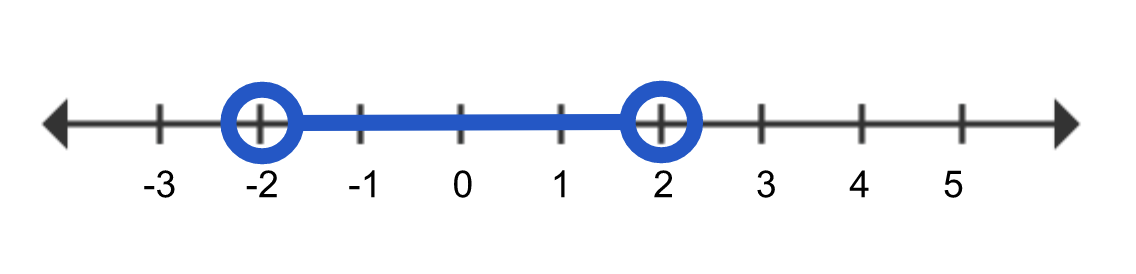
Another way to read this inequality would be the distance from zero is less than 2. So on a number line, we will shade all points that are less than 2 units away from zero.

This graph looks just like the graphs of the three-part compound inequalities! When the absolute value is less than a number we will remove the absolute value by changing the problem to a three-part inequality, with the negative value on the left and the positive value on the right. So in the example above, 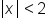becomes 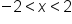, as the graph above illustrates, and can be expressed with the following formula:

formula to know

Absolute Value Inequalities - Less Than

big idea

If the absolute value inequality is "less than" or "less than or equal to", we can rewrite the inequality:

• Original inequality:
• Rewrite as:

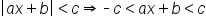
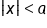
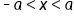
The inequality is a type of "AND" compound inequality.

2. "Greater Than" Absolute Value Inequalities

Let's look at a case when the absolute value inequality is "greater than".

EXAMPLE

Consider .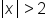

Absolute value is defined as the distance from zero. Another way to read this inequality would be the distance from zero is greater than 2. So on the number line, we shade all points that are more than 2 units away from zero.

This graph looks just like the graphs of the OR compound inequalities! When the absolute value is greater than a number we will remove the absolute value by changing the problem to an OR inequality, the first inequality looking just like the problem with no absolute value, the second flipping the inequality symbol and changing the value to a negative. So 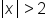becomes 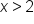or , as the graph above illustrates, and can be expressed with the following formula:

formula to know

Absolute Value Inequalities - Greater Than

big idea

If the absolute value inequality is "greater than" or "greater than or equal to", we can rewrite the inequality:

• Original inequality:
• Rewrite as:

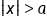
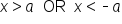
This inequality is a type of "OR" compound inequality.

try it

Rewrite each absolute inequality into inequalities you can solve.

Absolute Inequality	Rewrite as....

3. Solving Absolute Value Inequalities

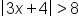
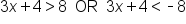
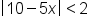
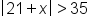
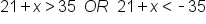
We can solve absolute value inequalities much like we solved absolute value equations by following these steps.

step by step

1. Make sure the absolute value is isolated on one side.
2. Remove the absolute value by either making a three-part inequality if the absolute value is less than a number, or making an OR inequality if the absolute value is greater than a number.
3. Solve the inequality.

hint

Remember, if we multiply or divide by a negative the inequality symbol will switch directions!

EXAMPLE

Solve the inequality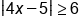 , graph the solution, and give interval notation of the solution.

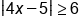
	Absolute value is greater than, use OR
	Solve by adding 5 to both sides in both inequalities
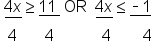
	Divide both sides by 4 in both inequalities
	Our Solution

Graph both inequalities.

Interval notation:

hint

For all absolute value inequalities, we can also express our answers in interval notation which is done the same way it is done for standard compound inequalities.

EXAMPLE

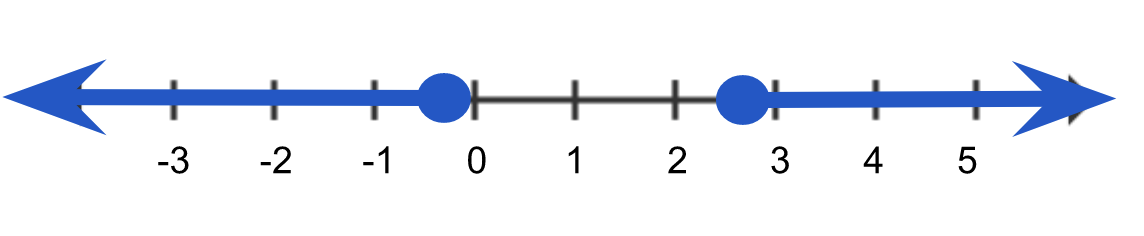
Solve the inequality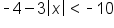 , graph the solution, and give interval notation of the solution.

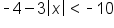
	First add 4 to both sides to isolate the absolute value
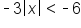
	Divide both sides by . Dividing by a negative switches the symbol!
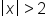
	Absolute value is greater than, use OR;
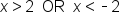
	Our Solution

Graph both inequalities.

Interval notation:

hint

In the previous example, we cannot combine -4 and -3 because they are not like terms, the -3 has an absolute value attached. So we must first clear the -4 by adding 4, then divide by -3. The next example is similar.

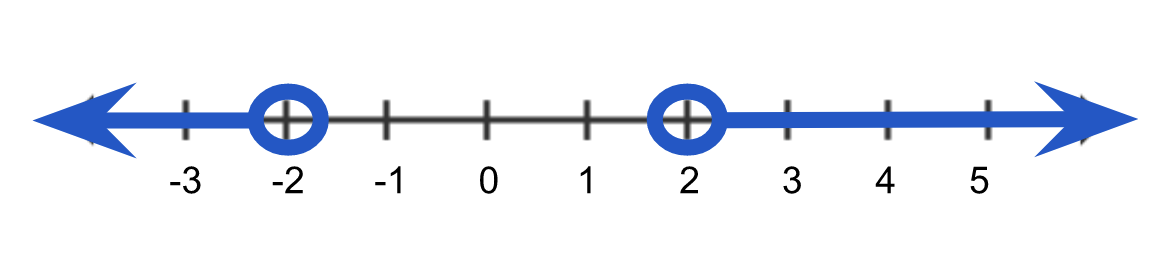
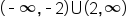
EXAMPLE

Solve the inequality , graph the solution, and give interval notation of the solution.

	Subtract 9 from both sides
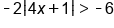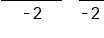
	Divide both sides by . Dividing by negative switches the symbol!
	Absolute value is less than, use three part inequality
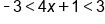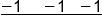
	Subtract 1 from all three parts
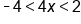
	Divide all three parts by 4
	Our solution

Graph both inequalities.

Interval notation:

hint

In the previous example, we cannot distribute the -2 into the absolute value. We can never distribute or combine things outside the absolute value with what is inside the absolute value. Our only way to solve is to first isolate the absolute value by clearing the values around it, then either make a compound inequality (either a three-part inequality or an OR inequality) to solve.

4. No Solutions or All Real Solutions

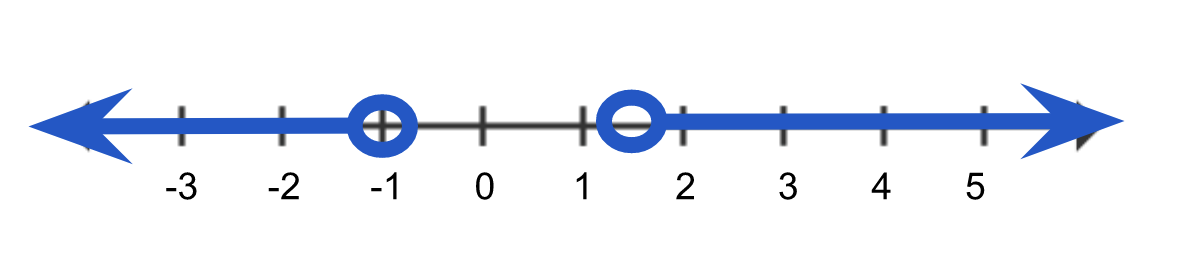
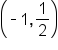
It is important to remember as we are solving these equations, the absolute value is always positive. There are cases where there is no solution to the inequality or all real numbers are the solution:

• No Solutions: If we end up with an absolute value is less than a negative number, then we will have no solution because absolute value will always be positive, greater than a negative.
• All Real Solutions: If the absolute value is greater than a negative, this will always happen. Here the answer will be all real numbers.

EXAMPLE

Solve the inequality , graph the solution, and give interval notation of the solution.

	Subtract 12 from both sides
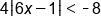
	Divide both sides by 4
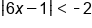
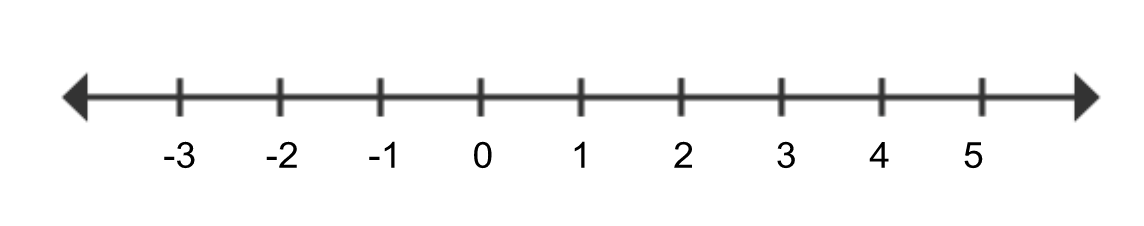
	Absolute value can't be less than a negative, NO SOLUTION

Graph showing no solution.

Interval notation: No Solution or

EXAMPLE

Solve the inequality , graph the solution, and give interval notation of the solution.

	Subtract 5 from both sides
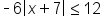
	Divide both sides by . Dividing by a negative flips the symbol!
	Absolute value is always greater than negative, ALL REAL NUMBERS

Graph showing all real numbers as solution.

Interval notation: All Real Numbers or