Solving a System of Linear Equations using Substitution

1. Solutions to a System of Equations

A solution to a system of linear equations is a specific coordinate pair (x, y) that satisfies all equations in the system. It is important that the solution satisfies every equation in the system, not just one; otherwise, it doesn't represent a solution to the entire system.

There are several ways to find solutions to a system of linear equations, such as by graphing or using the addition method. This lesson focuses on a different method known as the substitution method.

2. The Substitution Method

The main goal of the substitution method is to rewrite one of the equations so that one variable is isolated on one side of the equation, with everything else on the other side. As a result, that equivalent expression for the variable can be substituted into other equations in the system, in order to make solving for a particular variable possible. Once we find a value for one of the variables in a system, it becomes much easier to solve for other variables in the system.

Before we get into an example of using the substitution method to solve a system of equations, let's go over some general rules and tips.

It does not matter which equation or which variable you choose to isolate. As long as you don't make any algebraic errors, your answer will be the same no matter which route you choose. However, some choices are better than others, as they lower the chances of making algebraic errors. Keep these things in mind:

• Try to pick equations and variables that do not require fractions or complicated decimals. If you must, choose to work with simple fractions that you are familiar with.
• Variable terms with no coefficients are generally good choices when deciding which variable to isolate. This is because it will not require you to divide out the coefficient, which can lead to complicated fractions or decimals.
• Think about all of your options, and choose the option with the fewest calculations or steps.

EXAMPLE

Use the Substitution Method to find the solution to the following system of equations: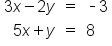

We have a couple of options as to how we should proceed. We need to choose one of the equations, and then re-write it so that it reads either or . Remember one of our helpful hints: choosing a variable term with no coefficient will reduce the number of steps, and make our calculations less complicated. For this reason, we are going to take the equation 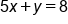, and rewrite it as . This will be the easiest because y is already isolated in this equation:

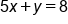
	Using the second equation, subtract 5x from both sides
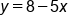
	An equivalent equation to the original

Now that we have an equivalent equation for y in terms of x, we want to turn our attention to the other equation we haven't use yet. When rewriting this equation, instead of writing y, we write the expression equivalent to y, which we just found to be :

	Using the first equation, substitute for y
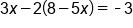
	Distribute -2 into
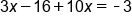
	Combine 3x and 10x
	Add 16 to both sides
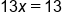
	Divide both sides by 13
	Our solution for x

Let's take a closer look at what happened above: by substituting our equivalent expression in for y, we eventually ended up with a single variable equation. Single-variable equations can be solved by applying inverse operations in order to isolate the variable. We have now found the value for x in our solution to the system. However, we still need to find the associated value for y.

To find y, we could plug 1 in for x in either of the two original equations in our solution. However, we can take a shortcut to solve for y. We already isolated y onto one side of an equation in our quest to solve for x. Let's use that equivalent equation to solve for y since much of the work has already been done.

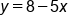
	Using the equivalent equation, plug 1 in for x
	Multiply 5 and 1
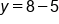
	Subtract 5 from 8
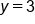
	Our solution for y

The solution to our system is (1, 3).

term to know

Substitution Method

A strategy for solving for variables in a system of equations by substituting variables for equivalent expressions.