Using FOIL to Represent Area

1. Representing Area with Binomial Multiplication

Recall that the area of a rectangle is found by multiplying the base times the height.

formula to know

Area of Rectangle

You can use this formula to solve real-world problems.

EXAMPLE

In the image below, we see a floor plan of an office space. In the office, there is the main section with workspaces and cubicles, a kitchenette, a break room, and a bathroom: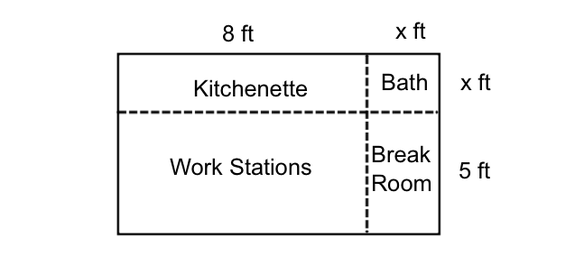



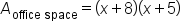
Note that we know some measurements of the areas in the office, while other measurements are unknown. Adding the partial dimensions together, we can express the area of the entire office using binomial multiplication.

We can expand this binomial multiplication using a process known as FOIL. FOIL stands for First, Outside, Inside, Last, and is used as an aid in remembering which terms to multiply together to expand two binomials being multiplied together.

Using FOIL, we see that we can equivalently express the area as:

	Multiply first terms:
	Multiply outside terms: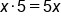
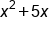
	Multiply inside terms:
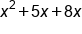
	Multiply outside terms:
	Expanded form

Notice that when we express the area using 4 terms, each term represents the area of the individual sections of the office:

• The bathroom has an area of square feet
• The kitchenette has an area of 8x square feet
• The break room has an area of 5x square feet
• The main work room has an area of 40 square feet

We can, and should, further simplify the area by combining like terms:

The area of the room can be expressed as .

2. Subtracting Area with Binomial Multiplication

We can also use the area equation to subtract space.

EXAMPLE

At a different office space, workers are coming in to install carpeting on the floor. However, they need to leave a border of wooden floors around the office for certain equipment. The floor plan is illustrated below:



We can represent the area of the carpet using binomial multiplication as well. However, our binomials will include subtraction, because we need to take away from the dimension of the office space. The area of the carpet can be expressed as:

We subtract 2x in this case because a distance of x feet is being trimmed from both sides of the length and width of the room.

Using FOIL, we can equivalently express the area as a polynomial in expanded form. This is illustrated below: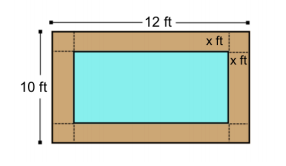

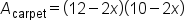
	Multiply first terms:
	Multiply outside terms:
	Multiply inside terms: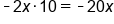
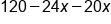
	Multiply last terms: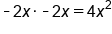
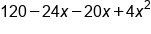
	Combine like terms
	Our solution

The area of the carpet can be expressed as .