Using Linear Equations in Real World Scenarios

1. Establishing a Linear Relationship

When establishing a linear relationship, you must first be given some information. This may be from a scenario, a graph, or sometimes even both are provided!

EXAMPLE

You are planning a road trip from St. Louis, Missouri to Las Vegas, Nevada. You look up the distance between the two cities, and estimate it to be 1600 miles. Since most of the trip will be on the open road, you also assume an average speed of 70 miles per hour.

We can think of the average speed of 70 miles per hour as the rate of change, or slope of a line when we graph this scenario. We also know that on a graph, we will have the point (0, 0) to represent the start of our trip, and the point (x, 1600) to represent the end of our trip: 1600 miles traveled after x number of hours.

Here is what the graph of our scenario looks like:

2. Writing a Linear Equation

We might be able to answer some questions related to our scenario by using the graph, although our answers might be approximate rather than exact, due to the scale on both the x- and y-axes. We can develop an equation for the line on the graph, and then use the equation to solve for exact answers algebraically.

EXAMPLE

Write a linear equation for the scenario and graph from above.

Perhaps using slope-intercept is the easiest form for the line we see on the graph. This is because we can easily see the y-intercept on the graph, which is at the point (0, 0). We also discussed earlier that the slope of the line is represented by the average speed of the car, 70 miles per hour.

Here is the equation for our line:

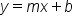
	Using slope intercept form, substitute the value of 0 in for b because the y-intercept is at (0, 0).
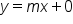
	Substitute the slope of 70 in for m
	Simplify
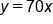
	Our solution

The linear equation for this scenario is .

3. Solving Problems Using the Equation of a Line

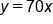Once you have an equation for a scenario, you can use this to answer some problems related to the situation.

EXAMPLE

The road trip is too much for one day. You figure that on your first day on the road, you can drive for about 6 hours. How many miles do you plan on driving on your first day?

To solve this problem, we substitute 6 in for x, because x represents hours. The corresponding y-value will represent miles traveled in 6 hours.

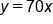
	Using our equation, substitute 6 in for x
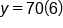
	Multiply 70 and 6
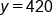
	Our Solution

This means you plan to travel 420 miles over 6 hours on your first day.

EXAMPLE

The total distance for your road trip is 1600 miles. How many hours will you spend on the road to get from St. Louis to Las Vegas?

To solve this problem, we will substitute 1600 in for y, because y represents miles. Solving for x will give us the time taken to travel 1600 miles.

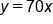
	Using our equation, substitute 1600 in for y
	Divide both sides by 70
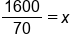
	Convert into a decimal
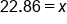
	Our solution (rounded)

This means it will take 22.86 hours (or about 22 hours and 52 minutes) to drive 1600 miles at an average speed of 70 miles per hour.