Table of Contents |
Logarithmic equations can be equivalently written using exponents. In general, we can say that the following two equations are equivalent:
| Exponential Equation | Logarithmic Equation |
|---|---|

|

|
Notice that the base of the logarithm is the base of the exponential expression. Additionally, the input of the logarithmic function is the output of the exponential function.
For some logarithmic equations, it may be helpful to rewrite the equation as an equivalent exponential equation to solve.
EXAMPLE
Solve the logarithmic equation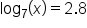 .
.
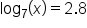
|
Rewrite into an exponential equation |

|
Evaluate using calculator |

|
Our solution |
EXAMPLE
Solve the logarithmic equation .
.

|
Divide both sides by 4 to have only 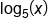 on left side on left side
|
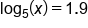
|
Rewrite into an exponential equation |

|
Evaluate using calculator |

|
Our solution |
Another method to solving log equations involves applying the inverse relationship between exponents and logs in a slightly different way than you may be used to. We can use the base of the logarithm as a base of an exponent and place the logarithmic expression as an exponent in the equation. We'll have to do this to both sides of the equation.
EXAMPLE
Solve the logarithmic equation .
.

|
Apply the base of the log, 3, as a base of an exponent on both sides. |
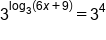
|
On the left side, the argument of the log  remains; on the right side, evaluate remains; on the right side, evaluate 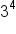
|
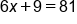
|
Subtract 9 from both sides |
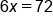
|
Divide both sides by 6 |
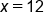
|
Our solution |
Source: ADAPTED FROM "BEGINNING AND INTERMEDIATE ALGEBRA" BY TYLER WALLACE, AN OPEN SOURCE TEXTBOOK AVAILABLE AT www.wallace.ccfaculty.org/book/book.html. License: Creative Commons Attribution 3.0 Unported License